Macroscopic physical interpretation

From these rather boring calculations we have learned several important lessons. We have shown that some scalar fields, which play a peculiar role in modern physics [106], can be generated in a purely geometric way.

We have also seen that we have to carefully choose the Lagrangian in order to get a consistent set of field equations that have a reasonably wide set of solutions. In fact, since the action principle (4.15) must be satisfied for an arbitrary choice of the integration surface $S$, one often obtains a too restrictive set of field equations. For instance, the choice of the matter Lagrangian is not independent of the choice of the geometric Lagrangian, since eq. (5.45) is necessary in order to avoid a contradiction between the normal equation (6.14) and the tangential equation (6.16)

Moreover, the functions $k, k_1,\ldots, k_5$ cannot be chosen at will, but they must satify eqs. (6.11), (6.17) and (6.19), which are not field equations, but just limitations to the form of the Lagrangian. According to these conditions, that are not independent, $k$, $k_4$ and $k_5$ can be chosen as arbitrary functions of $\phi$, but $k_3$ is uniquely determined and $k_1$, $k_2$ are determined up to an irrelevant additive constant, that adds to the Lagrangian an exact differential form.

We are interested in gravitational theories that do not contain the gravitational constant $G$ of Einstein's theory and we also require that they do not contain any other constant with nontrivial dimension (besides the velocity of light). Then $k$ has to be a power of $\phi$ and a possible numeric constant factor can be absorbed in the definition of the forms $\omega^{[ik]}$. Taking into account the above mentioned constraints, we put, as in ref. [5],

$$k = 2^{-2} \phi^{m - 1}, \qquad k_1 = 2^{-2} (m - 1)^{-1} \phi^{m - 1},$$

$$k_2 = 0, \qquad k_3 = 2^{-2} \phi^m, \qquad m \neq 1.$$ (6.25)

For $m = 1$, we have

$$k = 2^{-2}, \qquad k_1 = 2^{-2} \log(\phi / \phi_0), \qquad k_2 = 0 \qquad k_3 = 2^{-2} \phi,$$ (6.26)

where $\phi_0$ is a dimensional constant that, however, is irrelevant.

Other interesting results follow from a dimensional analysis. We indicate by $[L]$ the dimension of time and lenght and by $[M]$ the dimension of mass, energy and momentum. Since the coordinates of the manifold $\mathcal{S}$ are dimensionless, the Lagrangian form has the dimension of an action. We have the dimensional relations

$$[\lambda]= [LM], \qquad [\omega^i] = [L], \qquad [\chi_i] =[L^{-1}].$$ (6.27)

It follows from eqs. (5.1) that

$$[\omega^{[ik]}] = [\phi]^{-1} = [L^{-1/(2-m)}M^{1/(2-m)}], \qquad m \neq 2.$$ (6.28)

For $m = 2$ the introduction of a dimensional coupling constant is inavoidable, but this choice is already excluded by the conditions (6.11).

From eqs. (5.2) and (6.3) we also have

$$[k_4]= [L^{-3}M], \qquad [k_5] = [L^{-1}M]$$ (6.29)

and we have to put

$$k_5 = - \alpha \phi^{m - 2},$$ (6.30)

where $\alpha$ is a dimensionless constant. It is not possible to write $k_4$, that describes a cosmological term, as a function of $\phi$ without introducing a dimensional constant.

In order to write the field equations in a more familiar form, it is convenient to introduce the new vector fields

$$\tilde A_{[ik]} = \phi^{-1} A_{[ik]}, \qquad \tilde A_i = A_i.$$ (6.31)

As we have discussed in Section 2.2 and we shall see with more detail in the next Section 6.3, this change of basis in the tangent spaces $T_s \mathcal{S}$ may be operationally unjustified from a microscopic point of view.

We also define the new differential 1-forms

$$\tilde \omega^{[ik]} = \phi \omega^{[ik]}, \qquad \tilde \omega^i = \omega^i$$ (6.32)

and, by computing the commutators and taking into account the field equations (6.10), (6.12) and (6.20), we obtain the new structure coefficients

$$\tilde F_{[ik]j}^i = \phi^{-1} F_{[ik]j}^i = \hat F_{[ik]j}^i, \qqua... ...F_{[ik][jl]}^{[mn]} = \phi^{-1} F_{[ik][jl]}^{[mn]} = \hat F_{[ik][jl]}^{[mn]},$$

$$\tilde F_{[ik][jl]}^m = 0, \qquad \tilde F_{j[ik]}^{[mn]} = F_{j[ik]}^{[mn]} - \phi^{-1} A_j \phi (\delta^m_i \delta^n_k - \delta^n_i \delta^m_k) = 0.$$

$$\tilde F_{ik}^j = F_{ik}^j, \qquad \tilde F_{ik}^{[mn]} = \phi F_{ik}^{[mn]}.$$ (6.33)

They are compatible with a stucture of principal bundle of the manifold $\mathcal{S}$ and the fields $\tilde A_{[ik]}$ are the generators of the structural group namely of the Lorentz group.

We remark that, after the substitution (6.31), the field $\phi$ disappears from the Fermion Lagrangian (5.36) and we obtain a theory with a minimal coupling of the kind considered in Section 4.4. We also introduce the quantities

$$\tilde T_{[jk]}^i = \phi^{-1} T_{[jk]}^i,$$ (6.34)

that describe the usual angular momentum, corresponding to the infinitesimal transformations generated by $- \tilde A_{[jk]}$.

Expressed in terms of the new variables, the eqs. (6.13), (6.16), (6.21), take the form

$$2^{-1} \tilde F_{ik}^{[ik]} + \frac{d k_4}{d \Phi} - \alpha \chi_i \chi^i = 0,$$ (6.35)

$$\Phi F_{jk}^i + ( 1 -2 \alpha) \Phi (\chi_k \delta^i_j - \ch... ..._k \Phi \delta^i_j - A_j \Phi \delta^i_k) = \tilde T^i_{[jk]},$$ (6.36)

$$\Phi (- \tilde F_{jk}^{[ik]} + 2^{-1} \delta^i_j \tilde F_{lk}^{[lk]}) + 2 \alpha (\chi^i A_j \Phi - \chi^k A_k \Phi \delta^i_j)$$

$$+ 2 \alpha \Phi (A_j \chi^i - A_k \chi^k \delta^i_j) + (k_4 + \alpha \Phi \chi_k \chi^k) \delta^i_j = T^i_j,$$ (6.37)

where the field $\Phi$ given by

$$\Phi = \phi^{m - 2}$$ (6.38)

will be identified with the Brans-Dicke scalar field. After the change of basis (6.31), it has lost its geometric character since it cannot be written as a function of the new structure coefficients. The fields $\chi_i$ are still given by eq. (6.2). The locally measured gravitational constant is $G = (8 \pi \Phi)^{-1}$. In some treatments, the factor $8 \pi$ is included in the definition of $\Phi$ and it appears in the field equations.

Note that the dependence of the various coefficients on $\phi$ and in particular the exponent $m$ have disappeared from these equations. This means that the choice of the fundamental fields $A_{[ik]}$ is ambiguous in this macroscopic context, as we discuss in the next Section 6.3.

If we consider only Dirac spinning particles, from eq. (5.42) we see that eq. (6.36) is equivalent to the equations

$$F_{jk}^i + 3^{-1} (\chi_k \delta^i_j - \chi_j \delta^i_k) = \Phi^{-1} \epsilon^i{}_{jkl} W^l,$$ (6.39)

$$(2 - 6 \alpha) \chi_i - 3 \Phi^{-1} A_i \Phi = 0.$$ (6.40)

Assuming $\alpha \neq 1/3$, we have

$$F_{ki}^i = \chi_k = - \omega \Phi^{-1} A_k \Phi,$$ (6.41)

where

$$\omega = 3 (6 \alpha -2)^{-1}.$$ (6.42)

These equations show that the idea of replacing the derivatives of the scalar field by the vector part of torsion was correct.

We see from eq. (6.35) that, even in the absence of spinning particles, the torsion may not vanish. Since it does not appear in the Brans-Dicke equations, in order to compare them with our formulas, we have to eliminate torsion by changing again the choice of the fundamental vector fields, namely by introducing a torsionless connection, by means of the substitution

$$\breve A_k = A_k - 3^{-1} \chi^i \tilde A_{[ik]}, \qquad \breve A_{[ik]} = \tilde A_{[ik]}.$$ (6.43)

Note that, since $\Phi$ is a scalar field and $\chi_i$ is a vector field,

$$\breve A_k \Phi = A_k \Phi, \qquad \breve A_k \chi_i = A_k \chi_i + 3^{-1} \chi_k \chi_i - 3^{-1} \chi_j \chi^j g_{ik}.$$ (6.44)

By computing the Lie brackets of the new fields and using eq. (6.39) with $W^l = 0$, we obtain the new structure coefficients

$$\breve F_{jk}^i = 0,$$ (6.45)

$$\breve F_{jk}^{[il]} = \tilde F_{jk}^{[il]} - 3^{-2} \chi_m \chi^m (\delta_j^i \delta_k^l - \delta_k^i \delta_j^l)$$

$$- 3^{-1} (\breve A_j \chi^i \delta_k^l -\breve A_k \chi^i \delta_j^l - \breve A_j \chi^l \delta_k^i + \breve A_k \chi^l \delta_j^i)$$

$$+ 3^{-2} (\chi_j \chi^i \delta_k^l - \chi_k \chi^i \delta_j^l - \chi_j \chi^l \delta_k^i +\chi_k \chi^l \delta_j^i).$$ (6.46)

By means of these formulas and of eq. (6.41), we can write eqs. (6.35) and (6.37) in the form

$$- \breve F_{ik}^{[ik]} + 2 \omega \Phi^{-1} \breve A_j \brev... ... \breve A_j \Phi \breve A^j \Phi - 2 \frac{d k_4}{d \Phi} = 0,$$ (6.47)

$$\Phi (- \breve F_{kj}^{[ij]} + 2^{-1} \breve F_{jl}^{[jl]} \delta^i_k) - \breve A_k \breve A^i \Phi + \breve A_j \breve A^j \Phi \delta^i_k$$

$$- \omega \Phi^{-1}(\breve A_k \Phi \breve A^i \Phi - 2^{-1} \breve A_j \Phi \breve A^j \Phi \delta^i_k) + k_4 \delta^i_k = T^i_k$$ (6.48)

and, as a consequence, also taking eq. (6.41) into account, we obtain

$$(3 + 2 \omega) \breve A_i \breve A^i \Phi = T^i_i - 4 k_4 + 2 \Phi \frac{d k_4}{d \Phi}.$$ (6.49)

These are just the field equations of the Brans-Dicke theory [71], with the addition of a variable cosmological term.

The elimination of torsion by means of a different choice of the fundamental vector fields (namely of the connection) is not completely harmless, since the motion of spinning test particles may be influenced by torsion [37].

A comparison with astronomical measurements in the solar systems gives a rather high lower bound on the dimensionless parameter $\omega$. Recent data from Cassini-Huygens spacecraft give $\omega > 4 \times 10^4$ [109]. This means that $\alpha \approx 1/3$ and the field $\Phi$ is approximately constant. Then the limit $\omega \to \infty$ or $\alpha \to 1/3$ of the Brans-Dicke equations is physically very interesting, but it is not trivial [110].

In our geometric approach, however, we can introduce the choice $\alpha = 1/3$ from the begining directly in eq. (6.30), in the Lagrangian (6.3) and in eqs. (6.35), (6.37) and (6.39). From eq. (6.40) we see that $A_k \Phi = 0$, namely $\Phi = (8 \pi G)^{-1}$ is constant. From eq. (6.20) and (6.2) we also obtain

$$F_{l[jk]}^{[il]} = 0, \qquad \chi_i = F_{i \alpha}^{\alpha}.$$ (6.50)

In this way, we obtain a theory with a constant gravitational coupling, that is not determined by the theory, but by the initial conditions. It also has a dynamical torsion, since the quantities $\chi_i$, that represent the 4-vector part of the torsion, are true dynamical variables and their derivatives appear in the field equations. Theories with a dynamical torsion have been proposed by various authors.

If the spin density vanishes, by introducing the new fields $\breve A_{\alpha}$, we obtain the equations

$$\Phi (- \breve F_{kj}^{[ij]} + 2^{-1} \breve F_{jl}^{[jl]} \delta^i_k) + k_4 \delta^i_k = T^i_k$$ (6.51)

$$- 2 \Phi \breve A_i \chi^i = T^i_i - 4 k_4 + 2 \Phi \frac{d k_4}{d \Phi}.$$ (6.52)

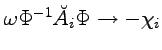These equations can also be obtained from the Brans-Dike equations (6.48) and (6.49) by introducing the substitution $\omega \Phi^{-1} \breve A_i \Phi \to - \chi_i$ before performing the limit $\omega \to \infty$.

The first formula is just the field equation of general relativity. The second formula determines only partially the vector field $\chi_i$ that, since torsion has been eliminated, has lost its geometric meaning and does not give any contribution to the source of the gravitational field. These properties of $\chi_i$ are hardly acceptable from the physical point of view. One may suggest that the model in not complete and that new terms have to be added to the Lagrangian. We shall consider again this suggestion in Chapter 7.