Index

(10 + n)-momentum

Flat Minkowski spacetime and

10-momentum

Flat Minkowski spacetime and

absolute parallelism

The basic geometric and

action principle

The action principle and

active transformations

Tensor and spinor fields | Flat Minkowski spacetime and | Noether's theorem

anholonomic components

Tetrads

anti-de Sitter spacetime

Flat Minkowski spacetime and

axial symplectic subgroup

Subgroups of $SL(4, \mathbf{R})$

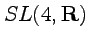
balance equation

Minimal coupling and the

base of a bundle

Tetrads

bundle of extended frames

Maxwell and Yang-Mills fields

Cabibbo-Kobayashi-Maskawa matrix

Fermions in the Standard

canonical form

Connection, soldering, curvature and

causal influence

Orbits in $\mathcal{T}$ and

causally disjoint

Orbits in $\mathcal{T}$ and

charges

Flat Minkowski spacetime and

classical geometry

Critical remarks

complete solution

Dynamical variables and symmetry

composition of transformations

The operational interpretation and

cone

A wedge 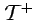$\mathcal{T}^+$ in

connected solution

Dynamical variables and symmetry

connection

A compact formalism

connection coefficients

Parallel transport

connection form

Connection, soldering, curvature and

constant solution

Dynamical variables and symmetry

contraction of groups

Subgroups of $SL(4, \mathbf{R})$

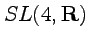
cosmological constant

The Einstein-Cartan theory of

current

Conserved forms

curvature form

Connection, soldering, curvature and

de Sitter spacetime

Flat Minkowski spacetime and

diffeomorphisms

Dynamical variables and symmetry

dimensional analysis

Macroscopic physical interpretation

Dirac brackets

Pre-symplectic formalism and double

Dirac's large numbers problem

A geometrized scalar-tensor theory

distinguished point

Flat Minkowski spacetime and | Normal field equations and

double differential forms

Pre-symplectic formalism and double

dynamical torsion

Macroscopic physical interpretation

energy-momentum

Flat Minkowski spacetime and

equity principle

The equity principle, the

events

The spacetime coincidence

extremal elements

The Lorentz invariant cone

feasibility cone

The Lorentz invariant cone

feasible infinitesimal transformations

A wedge 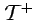$\mathcal{T}^+$ in

fiber

Tetrads

foliation

The spacetime coincidence

fundamental vector fields

The basic geometric and | The operational interpretation and

gauge field strength

Parallel transport

gauge field theories

Tetrads

gauge fixing constraints

Pre-symplectic formalism and double

gauge transformation

Dynamical variables and symmetry

generalized Gauss law

Conserved forms

geometric symmetry group

Dynamical variables and symmetry

global spacetime interpretation

The spacetime coincidence

Hausdorff separation axiom

The spacetime coincidence

holonomic components

Tetrads

homogeneous space

Dynamical variables and symmetry

horizontal degree

A compact formalism

horizontal subpace

A compact formalism

horizontal tangential equations

The Einstein-Cartan theory of

ideal accelerated clock

The symmetry group $GL(4, \mathbf{R})$

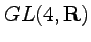
ideal clock

The symmetry group $GL(4, \mathbf{R})$

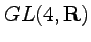
infinitesimal transformations

The operational interpretation and

integrable distribution

The spacetime coincidence

integral manifolds

The spacetime coincidence

internal degree

A compact formalism

internal gauge theory

Internal gauge theories

Lagrangian form

The action principle and

leaf

The spacetime coincidence

left translations

Flat Minkowski spacetime and

Lie derivative

Dynamical variables and symmetry

local section

Covariant derivatives and spin

local spacetime interpretation

The spacetime coincidence

Lorentz vertical degree

A compact formalism

Mack's principle

Microscopic considerations and dilatations

Maurer-Cartan one-forms

Maxwell and Yang-Mills fields

Maxwell 2-form

Conserved forms

Maxwell equations

Internal gauge theories

measurement

The operational interpretation and

measurement procedures

The operational interpretation and

metricity condition

Parallel transport

minimal coupling

Minimal coupling and the

minimum time principle

The equity principle, the

Minkowski spacetime

Flat Minkowski spacetime and

modified geometry

The Lorentz invariant cone

moving frame

Tetrads

normal field equations

The action principle and

normal geometry

The basic geometric and

normal limit

Higher symmetries and a

orientable manifold

Tetrads

parallel transport

Parallel transport

passive transformations

Tensor and spinor fields | Flat Minkowski spacetime and

phase space

Pre-symplectic formalism and double

physical equivalence

The spacetime coincidence

pre-symplectic space

Pre-symplectic formalism and double

preparation procedure

Critical remarks

primary Lagrangian constraints

Pre-symplectic formalism and double

primary local spacetime coincidence

The spacetime coincidence

principal fiber bundle

Tetrads

procedures

The operational interpretation and

projection mapping

Tetrads

pseudo-Finslerian space

The symmetry group $GL(4, \mathbf{R})$

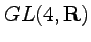
pseudo-norm

The symmetry group $GL(4, \mathbf{R})$

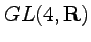
reciprocal influence

Orbits in $\mathcal{T}$ and

regular cubic coordinate system

The spacetime coincidence

relational geometry

The operational interpretation and

relativistic angular momentum

Flat Minkowski spacetime and

relativity principle

The operational interpretation and

repère mobile

Tetrads

restricted measurement procedures

Orbits in $\mathcal{T}$ and

restricted measurements

Orbits in $\mathcal{T}$ and

reversible infinitesimal transformations

A wedge 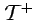$\mathcal{T}^+$ in

Riemann curvature tensor

Parallel transport

right translations

Flat Minkowski spacetime and

scalar field

Scalar fields

scalar-tensor theory

A geometrized scalar-tensor theory

secondary local spacetime coincidence

The spacetime coincidence

situation

The operational interpretation and

soldering form

Connection, soldering, curvature and

space of motions

Pre-symplectic formalism and double

spacelike submanifold

Orbits in $\mathcal{T}$ and

spacetime coincidence

The spacetime coincidence

spatially localized quantity

Conserved forms

spin connection coefficients

Covariant derivatives and spin

spin structure

Tensor and spinor fields

spinor

Tensor and spinor fields

spinors

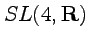$SL(4, \mathbf{R})$ spinors and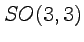 $SO(3,3)$

spontaneous symmetry breaking

The spinor representation of

Standard Model

Fermions in the Standard

strictly operational interpretation

The operational interpretation and

structural group

Tetrads

structure coefficients

A compact formalism

structure equations

Connection, soldering, curvature and

subsolution

Dynamical variables and symmetry

substitution rule

Higher symmetries and a

symplectic double form

Pre-symplectic formalism and double

symplectic form

Pre-symplectic formalism and double

tangential field equations

The action principle and

teleparallelism

The basic geometric and

tetrad

Tetrads

time orientable manifold

Tetrads

torsion form

Connection, soldering, curvature and

torsion tensor

Parallel transport

total degree

A compact formalism

total dilatations

Microscopic considerations and dilatations

transformation procedures

The operational interpretation and

transformations

The operational interpretation and

trivialization

The basic geometric and

vacuum state

Dynamical variables and symmetry

vector symplectic subgroup

Subgroups of $SL(4, \mathbf{R})$

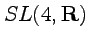
vertical reflection

Subgroups of $SL(4, \mathbf{R})$

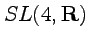
vertical subpace

A compact formalism

vertical tangential equations

The Einstein-Cartan theory of

Vierbein

Tetrads

wedge

A wedge 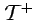$\mathcal{T}^+$ in